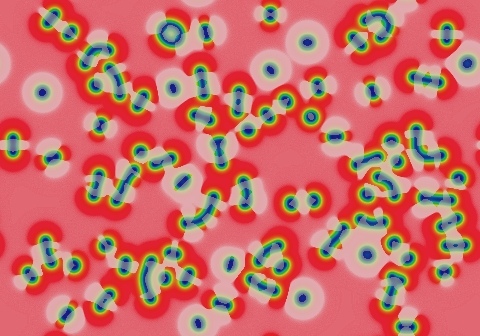
Gray-Scott Model at F 0.0700, k 0.0630

These images and movie demonstrate the behavior of the Gray-Scott reaction-diffusion system with σ=D_u/D_v=2 and parameters F=0.0700, k=0.0630.

Worms grow to fill the space; loops change to worms with relatively little pressure; solitons also turn to worms but can remain stable if confined. Branching structures are also viable (lower right). At 0:45 in the movie we see a worm (just above center) get squeezed back into a soliton. Pattern develops towards parallel lines and 60^o angles.

The eastern limit for worms at F=0.0700 is around k=0.0644, beyond which they shrink to solitons.

Categories: Pearson μ; Wolfram 2-a (glossary of terms)

	increase F
decrease k	15 frames/sec.; each fr. is 216 iter. steps = 108 tu; 1800 fr. total (194,400 tu)	increase k

	decrease F

(Click on any image to magnify)

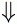In these images:

Color indicates level of u, ranging from purple (lowest u values) through blue, aqua, green, yellow and pink/red (highest u values)
Areas where u is increasing are lightened to a light pastel tone; where u is decreasing the color is vivid.
In areas where u is changing by less than ±3×10^-6 per tu, an intermediate pastel color is seen. This includes areas that are in steady state or equilibrium.

Wavefronts and other moving objects have decreasing u values (brighter color) on the leading edge of the blue part of the moving object, and increasing u (light pastel color) on the trailing edge. This is true even for very slow-moving objects — thus, you can tell from the coloring what direction things are moving in.

''tu'' is the dimensionless unit of time, and ''lu'' the dimensionless unit of length, implicit in the equations that define the reaction-diffusion model. The grids for these simulations use Δx=1/143 lu and Δt=1/2 tu; the system is 3.2 lu wide. The simulation meets itself at the edges (periodic boundary condition); all images tile seamlessly if used as wallpaper.

Go back to Gray-Scott pattern index

This page was written in the "embarrassingly readable" markup language RHTF, and was last updated on 2019 Jan 05.

s.11