Radar Basics

James Clerk Maxwell

James Clerk Maxwell (13 June 1831 – 5 November 1879) was a Scottish scientist in the field of mathematical physics. He became famous for his mathematical description of the magnetic and electric fields known as Maxwell's equations.

Maxwell relied on Michael Faraday's experimentally discovered connections between the movement of a magnet and the voltage induced by it in an electrical conductor, the later law of induction. Faraday spoke of single lines of force which the moving magnet draws behind it. Maxwell, on the other hand, imagined that the entire space around the magnet was filled with a force field. Complicated differential equations were required to calculate such a force field. He also tried to find the laws which determine the behaviour of any system of electrically charged particles when their present position and velocity and the value of the field are given at all its points.

After achieving this, he found that Faraday's experimentally found laws could not be complete, that the equations were ambiguous and did not lead to any particular result. Solution was to assume, not only moving magnet produces electric field (and thus electric current within conductor), but also moving electric charge produces very small magnetic field. If one assumes this, one can create complete, unambiguous equations.¹

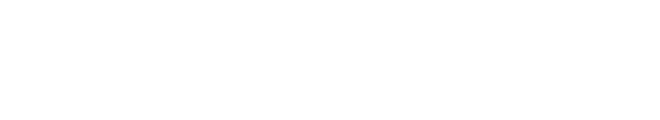
• Equation 1: The distribution of an electric charge is the cause of an electric field. The electric flow through the closed surface ∂ V of a volume V is directly proportional to the electric charge inside it.

• Equation 2: Magnetic field lines form closed circles. The magnetic flux through the closed surface of a volume is equal to the magnetic charge in its interior (that is zero, since there are no magnetic monopoles).

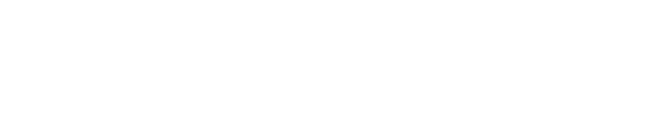
• Equation 3: A time-varying magnetic field generates an electric field. The electric circulation over the boundary curve ∂ A of a surface A is equal to the negative temporal change of the magnetic flux through the surface.

• Equation 4: A moving charge or an alternating electric flux both generate a magnetic field. The magnetic circulation over the boundary curve ∂ A of a surface A is equal to the sum of the (electric) current and the temporal change of the electric flux through the surface.

One of the most surprising results of these equations was that electric fields and magnetic fields can be caused to wave. These wave movements have a precisely defined speed, which depends on the strength of the electric field generated by a moving magnet. This means than that the desired speed of the waves corresponds to the speed that a magnet must be given if the electric field generated is to have the same energy as its own magnetic field. This speed was already known and Maxwell pointed out that it was in best agreement with the speed attributed to light at the time. He therefore asserted that the light waves were of electromagnetic origin.¹

These purely mathematical equations thus predict the existence of electromagnetic waves. Their existence was confirmed 20 years later by experiments by Heinrich Rudolf Hertz and are the basis of the entire radio and radar technology.

Nowadays the Maxwell equations are no longer used in this complicated integral notation. The so-called Nabla notation (mathematical character: ∇ ) is preferred.