What is the radar range equation?
The Radar Range Equation
The radar range equation represents the physical dependences of the transmit power, which is the wave propagation up to the receiving of the echo signals. The power PE returning to the receiving antenna is given by the radar equation, depending on the transmitted power PS, the slant range R, and the reflecting characteristics of the aim (described as the radar cross-section σ). At the known sensibility of the radar receiver, the radar equation determines the achieved by a given radar theoretically maximum range. Furthermore one can assess the performance of the radar set with the radar range equation (or shorter: the radar equation).
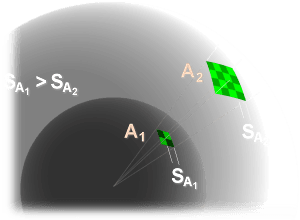
Figure 1: Nondirectional power density diminishes as the geometric spreading of the beam.
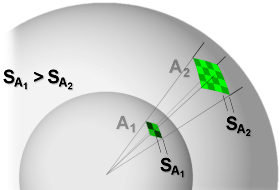
Figure 1: Nondirectional power density diminishes as the geometric spreading of the beam.
Argumentation/Derivation
First, we assume, that electromagnetic waves propagate under ideal conditions, i.e. without dispersion.

Figure 1: Nondirectional power density diminishes as geometric spreading of the beam.
If high-frequency energy is emitted by an isotropic radiator then the energy propagates uniformly in all directions. Areas with the same power density, therefore, form spheres (A= 4 π R²) around the radiator. The same amount of energy spreads out on an incremented spherical surface at an incremented spherical radius. That means: the power density on the surface of a sphere is inversely proportional to the square of the radius of the sphere.
So we get the equation to calculate the Non-directional Power Density Su
.print.png)
.png)
(1)
- PS = transmitted power [W]
- Su = nondirectional power density
- R1 = range from transmitter antenna to the aim [m]
Since a spherical segment emits equal radiation in all direction (at constant transmit power) if the power radiated is redistributed to provide more radiation in one direction, then this results in an increase of the power density in direction of the radiation. This effect is called antenna gain. This gain is obtained by directional radiation of the power. So, from the definition, the directional power density is:
(2)
- Sg = directional power density
- Su = nondirectional power density
- G = antenna gain
Of course, in reality, radar antennas aren't “partially radiating” isotropic radiators. Radar antennas must have a small beamwidth and an antenna gain up to 30 or 40 dB. (e.g. parabolic dish antenna or phased array antenna).
The target detection isn't only dependent on the power density at the target position, but also on how much power is reflected in the direction of the radar. In order to determine the useful reflected power, it is necessary to know the radar cross-section σ. This quantity depends on several factors. But it is true to say that a bigger area reflects more power than a smaller area. That means:
An Airbus offers more radar cross-section than a sporting aircraft at the same flight situation. Beyond this the reflecting area depends on the design, surface composition and materials used.
If the previously mentioned is summarized, the reflected power Pr (at the destination, i.e. the radar receiver) results from the power density Su, the antenna gain G, and the very variable reflection area σ:
.print.png)
.png)
(3)
- Pr = reflected power [W]
- σ = radar cross-section [m²]
- R1 = range, distance antenna - aim [m]
Simplified a target can be regarded as a radiator in turn due to the reflected power. In this case, the reflected power Pr is the emitted power.
Since the echoes encounter the same conditions as the transmitted power, the power density yielded at the receiver Se is given by:

Figure 2: Connection between
equations (3) and (4)
Figure 2: Connection between
equations (3) and (4)
.print.png)
.png)
(4)
- Se = power density at receiving place
- Pr = reflected power [W]
- R2 = range aim - receiving antenna [m]
At the radar antenna, the received power PE is dependent on the power density at the receiving site se and the effective antenna aperture AW.
(5)
- PE = received power [W]
- AW = effective antenna aperture [m²]
The effective antenna aperture arises from the fact that an antenna suffers from losses, therefore, the received power at the antenna is not equal to the input power. As a rule, the efficiency of the antenna is around 0.6 to 0.7 (Efficiency Ka).
Applied to the geometric antenna area, the effective antenna aperture is:
(6)
- AW = effective antenna aperture [m²]
- A = geometric antenna area [m²]
- Ka = efficiency
The power received, PE is then calculated:
.png)
.print.png)
.png)
The transmitted and reflected waves have been seen separately. The next step is to consider both transmitted and reflected power: Since R2 (aim - antenna) is equal to the distance R1 (antenna - aim) then
.png)
.print.png)
.png)
(9)
Another equation, which will not be derived here, describes the antenna gain G in terms of the wavelength λ.
.print.png)
.png)
(10)
Solving for A, antenna area, and replacing A into equation 9; after simplification it yields:
.print.png)
.png)
(11)
Solving for range R, we obtain the classic radar equation:
.print.png)
.png)
(12)
All quantities that influence the wave propagation of radar signals were taken into account at this equation. Before we attempt to use the radar equation in the practice for example to determine the efficiency of radar sets, some further considerations are necessary.
For given radar equipment most sizes (Ps, G, λ) can be regarded as constant since they are only variable parameters in very small ranges. The radar cross-section, on the other hand, varies heavily but for practical purposes, we will assume 1 m².
The smallest received power that can be detected by the radar is called PEmin. Smaller powers than PEmin aren't usable since they are lost in the noise of the receiver. The minimum power is detected at the maximum range Rmax as seen from the equation.
.print.png)
.png)
(13)
An application of this radar equation is to easily visualize how the performance of the radar sets influences the achieved range.
Influences on the Maximum Range of a Radar Set
All considerations, when calculating the radar equation, were made assuming that the electromagnetic waves propagate under ideal conditions without disturbing influences. In practice, a number of losses should be considered since they reduce the effectiveness of the radar considerably.
First, the radar equation is extended by including the loss factor Lges.
.print.png)
.png)
(14)
This factor includes the following losses:
- L D = internal attenuation factors of the radar set on the transmitting and receiving paths
- L f = fluctuation losses during the reflection
- L Atm = atmospheric losses during propagation of the electromagnetic waves to and from the target
High-frequency components, such as waveguides, filters and also a radome, generate internal losses. For a given radar set this loss is relatively constant and also easily measured.
Atmospheric attenuation and reflections at the Earth's surface are permanent influences.
Influence of the Earth's Surface
An extended but less frequently used form of the radar equation considers additional terms, like the Earth's surface but does not classify receiver sensitivity and atmospheric absorption.
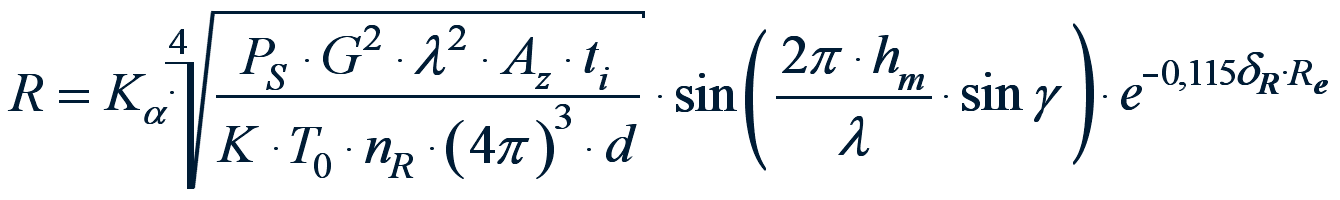
(15)
In this equation, in addition to the already well-known quantities are:
| Kα | = Loss factor in place of Lges. | Az | = Effective reflection surface in place of σ |
| ti | = Pulse length | K | = Boltzmann's constant |
| T0 | = absolute temperatur in K | nR | = Noise figure of the receiver |
| d | = Clarity factor of the display terminal | γ | = Reflected beam angle |
| δR | = Break-even factor | Re | = Distance of the absorbing medium |
Radar Reflections from Flat Ground
The trigonometric representation shows the influence of the Earth's surface.
The Earth plane surrounding radar antenna has a significant impact on the vertical polar diagram.
The combination of the direct and re-reflected ground echo changes the transmitting and
receiving patterns of the antenna. This is substantial in the VHF range and decreases with
increasing frequency. For the detection of targets at low heights, a reflection at the
Earth's surface is necessary. This is possible only if the ripples of the area within the first
Fresnel zone
do not exceed the value 0.001 R (i.e.: Within a radius of 1000 m no obstacle may
be larger than 1 m!).
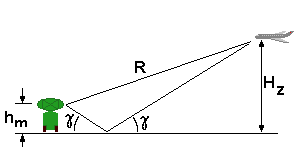
Figure 3: Detour of ground reflections
Figure 3: Detour of ground reflections
Specialized Radars at lower (VHF-) frequency band make use of the reflections at the Earth's surface and lobing to maximize cover at low levels. At higher frequencies, these reflections are more disturbing. The following picture shows the lobe structure caused by ground reflections. Normally this is highly undesirable as it introduces intermittent cover as aircraft fly through the lobes. The technique has been used in ATC ground mounted radars to extend the range but is only successful at low frequencies where the broad lobe structure permits adequate cover at higher elevations.
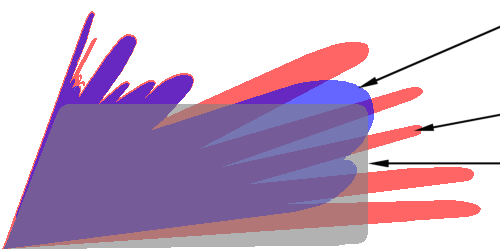
here it is the idealized cosecant-squared- diagram!
Figure 4: A vertical pattern diagram with influences of ground reflections

here it is the idealized cosecant-squared- diagram!
Figure 4: A vertical pattern diagram with influences of ground reflections
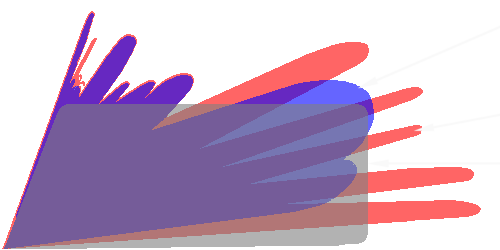
here it is the idealized cosecant-squared- diagram!
Figure 4: A vertical pattern diagram with influences of ground reflections
Increasing the height of the antenna has the effect of making finer the lobing pattern. A fine-grained lobing structure is often filled in by irregularities in the ground plane. Specifically, if the ground plane deviates from a flat surface then the reinforcement and destruction pattern resulting from the ground reflections breaks down. Avoidance of lobe effects is one of the prime considerations when selecting radar location and the height of the antenna.
