Fluctuation Loss
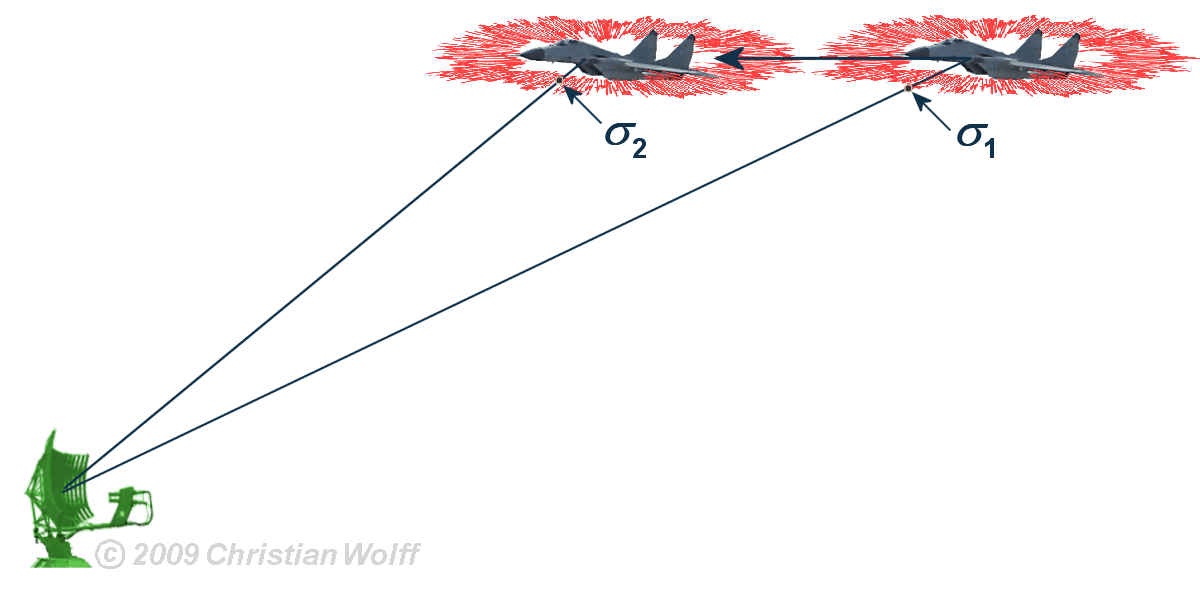
Figure 1: the rotating diagram as the cause of fluctuation
Fluctuation Loss
The fluctuation of the reflected signal is based on the complicated diagram of the relative radar cross-section (RCS). At a forward movement the RCS diagram of the airplane is turned in the reference to the radar set. Caused by the temporal changes of the aim course the amplitudes and phase changes effect a strong fluctuation of the reception field strength at the radar antenna.
Swerling target models are special cases of the Chi-Square target models with specific degrees of freedom.
The Swerling models were introduced in 1954 by the American mathematician Peter Swerling and are used to describe the statistical properties of the radar cross-section of objects with complex formed surface. According to the Swerling models the RCS of a reflecting object based on the chi-square probability density function with specific degrees of freedom. These models are of particular importance in the theoretically radartechnology. There are five different Swerling models, numbered with the Roman numerals I through V:
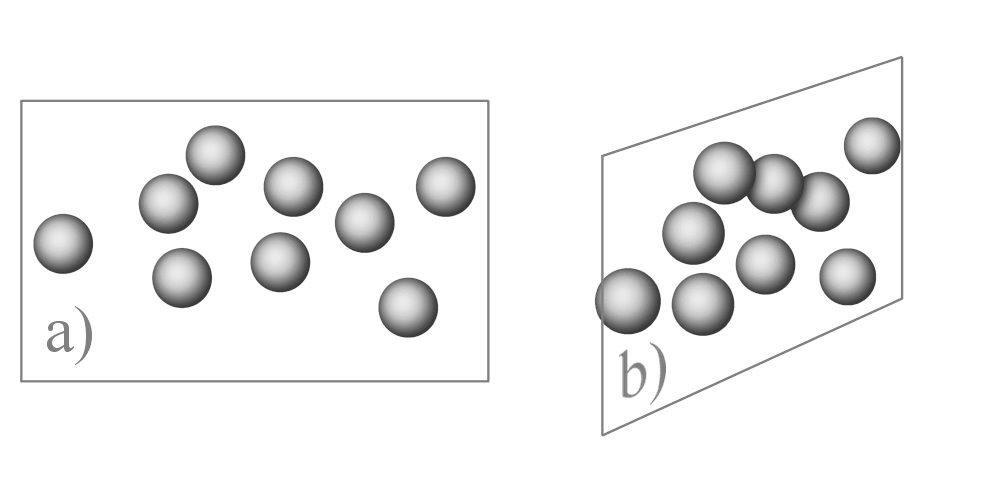
Figure 2: Swerling I and II: The target consists of a number of equally large isotropic reflectors which are distributed on a surface. Another aspect angle of the same assembly (view b) results to other distances and thus to other interferences.

Figure 2: Swerling I and II: The target consists of a number of equally large isotropic reflectors which are distributed on a surface. Another aspect angle of the same assembly (view b) results to other distances and thus to other interferences.
Swerling I Target
This case describes a target whose magnitude of the backscattered signal is relatively constant during the dwell time. It varies according to a Chi-square probability density function with two degrees of freedom (m = 1). The radar cross-section is constant from pulse-to-pulse, but varies independently from scan to scan. The density of probability of the RCS is given by the Rayleigh-Function:
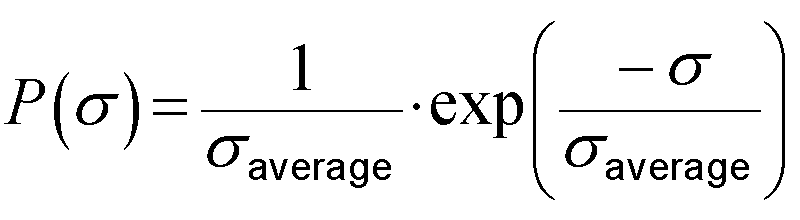
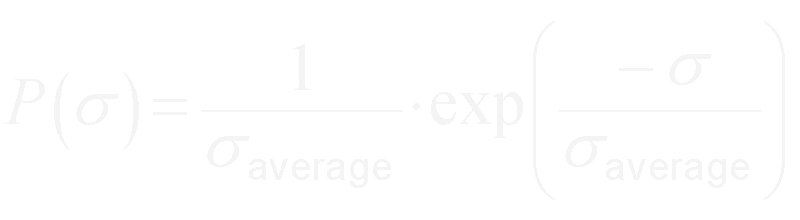 (44)
(44)Where σaverage is the arithmetic mean of all values of RCS of the reflecting object.
Swerling II Target
The Swerling II target is similar to Swerling I, using the same equation, except the RCS values changes faster and varies from pulse to pulse additionally.
The Swerling cases I and II applies to a target that is made up of many independent scatterers of roughly equal areas like airplanes. However, in Swerling case II there is no rotating surveillance antenna but a focused onto a target tracking radar.
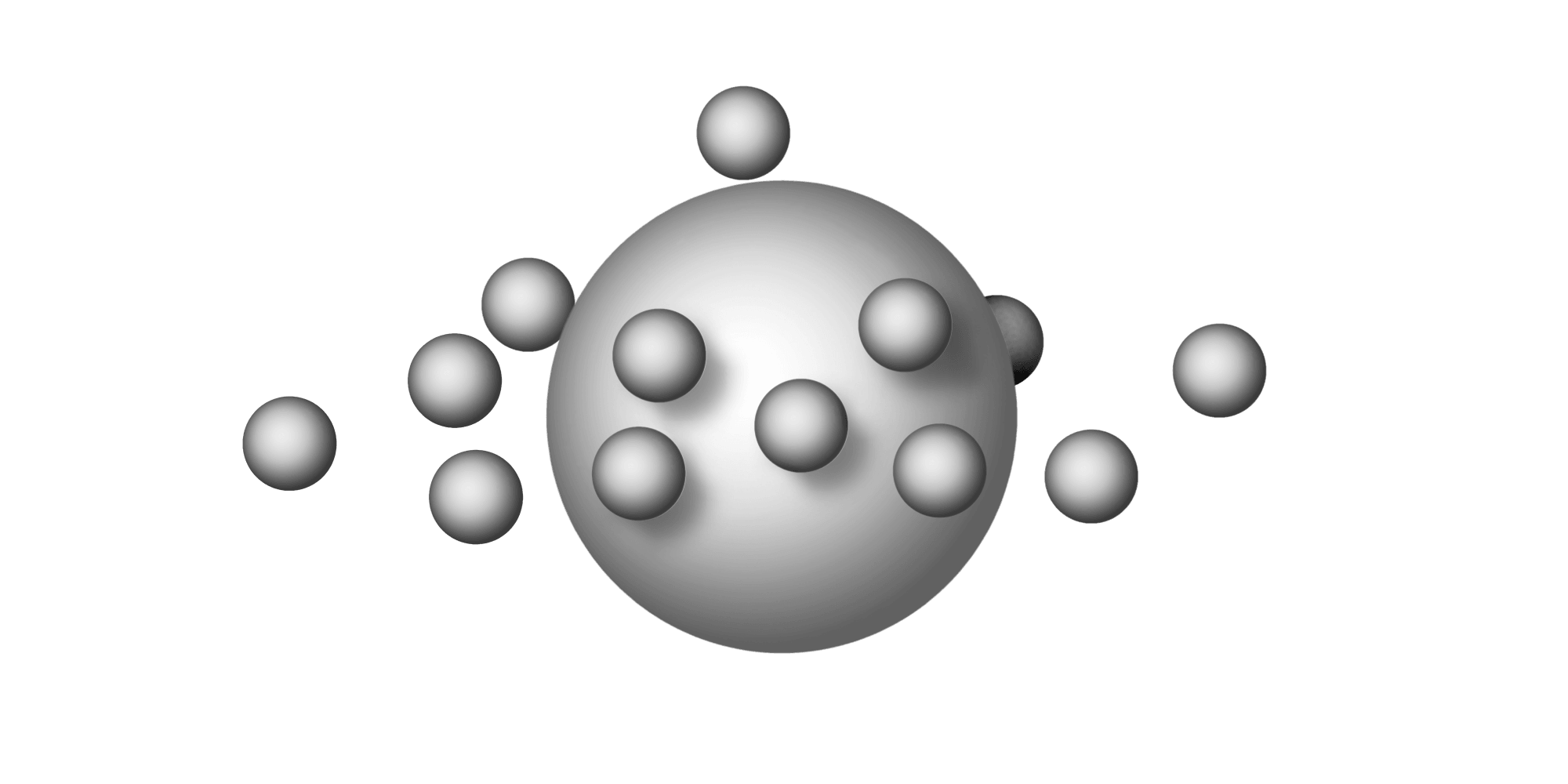
Figure 3: Swerling III and IV: A dominant isotropic reflector is superimposed by a plurality of small reflectors.

Figure 3: Swerling III and IV: A dominant isotropic reflector is superimposed by a plurality of small reflectors.
Swerling III Target
The Swerling III target is decribed like Swerling I but with four degrees of freedom (m = 2). The scan-to-scan fluctuation follows a density of probability:
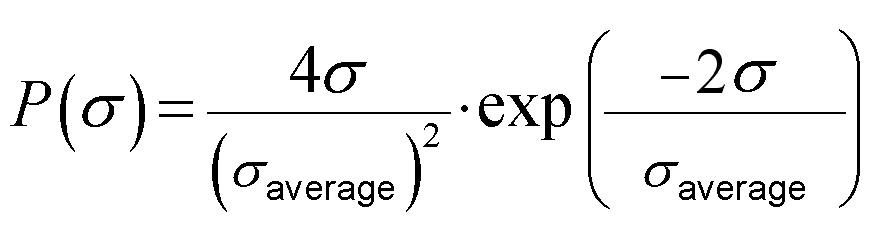
 (45)
(45)Swerling IV Target
The Swerling case IV is similar to Swerling III but the RCS varies from pulse to pulse rather than from scan to scan and follows the Eq. 45.
Cases III and IV approximates an object with one large scattering surface with several other small scattering surfaces. This may be the case for ships. Swerling shows in his publication, that an additional fluctuation loss depends more on the probability of detection and less on the probability of false alarms PN.
Given values of the theoretically maximum range of a tracking radar set are based on the Swerling II and IV Target Model often. The fluctuation loss of a steady target is with the typical value of 1 to 2 Decibels relatively small at a probability of detection PD=60%.
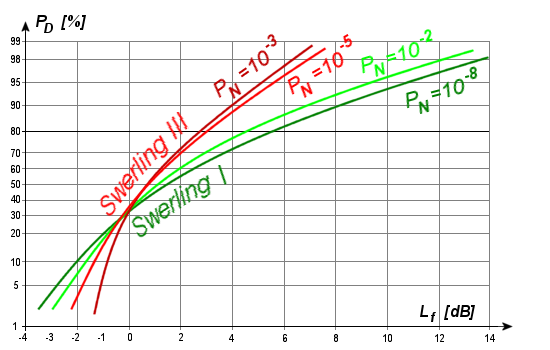
Figure 2: Fluctuation loss Lf for the Swerling cases I and III

Figure 2: Fluctuation loss Lf for the Swerling cases I and III
The cases I and III apply for search radars. The fluctuation loss depends on the probability of detection and is shown in Figure 1. There is a fluctuation gain for a PD<30%. This is while the statistically changing of the magnitude excels small signal-to-noise ratios.
Swerling V
The Swerling case V is a reference value with a constant radar cross-section (also known as Swerling 0). It describes an idealized target without any fluctuation.
Source:
- Swerling: ''Probability of Detection for Fluctuating Targets'' Rand Research Memorandum RM-1217, March 17, 1954