Radars Accuracy
Accuracy is the degree of conformance between the estimated or measured position and/or the velocity of a platform at a given time and its true position or velocity. Radio navigation performance accuracy is usually presented as a statistical measure of system error and is specified as:
- Predictable: The accuracy of a position in relation to the geographic or geodetic coordinates of the earth.
- Repeatable: The accuracy in which a user can return to a position whose coordinates have been measured at a previous time with the same navigation system.
- Relative: The accuracy which a user can determine one position relative to another (by neglecting all possible errors).
The stated value of required accuracy represents the uncertainty of the reported value with respect to the true value and indicates the interval in which the true value lies with a stated probability. The recommended probability level is 95 percent, which corresponds to 2 standard deviations of the mean for a normal (Gaussian) distribution of the variable. The assumption that all known correction is taken into account implies that the errors in the reported values will have a mean value (or bias) close to zero.
Any residual bias should be small compared with the stated accuracy requirement. The true value is that value which, under operational conditions, characterizes perfectly the variable to be measured/observed over the representative time, area and/or volume interval required, taking into account siting and exposure.
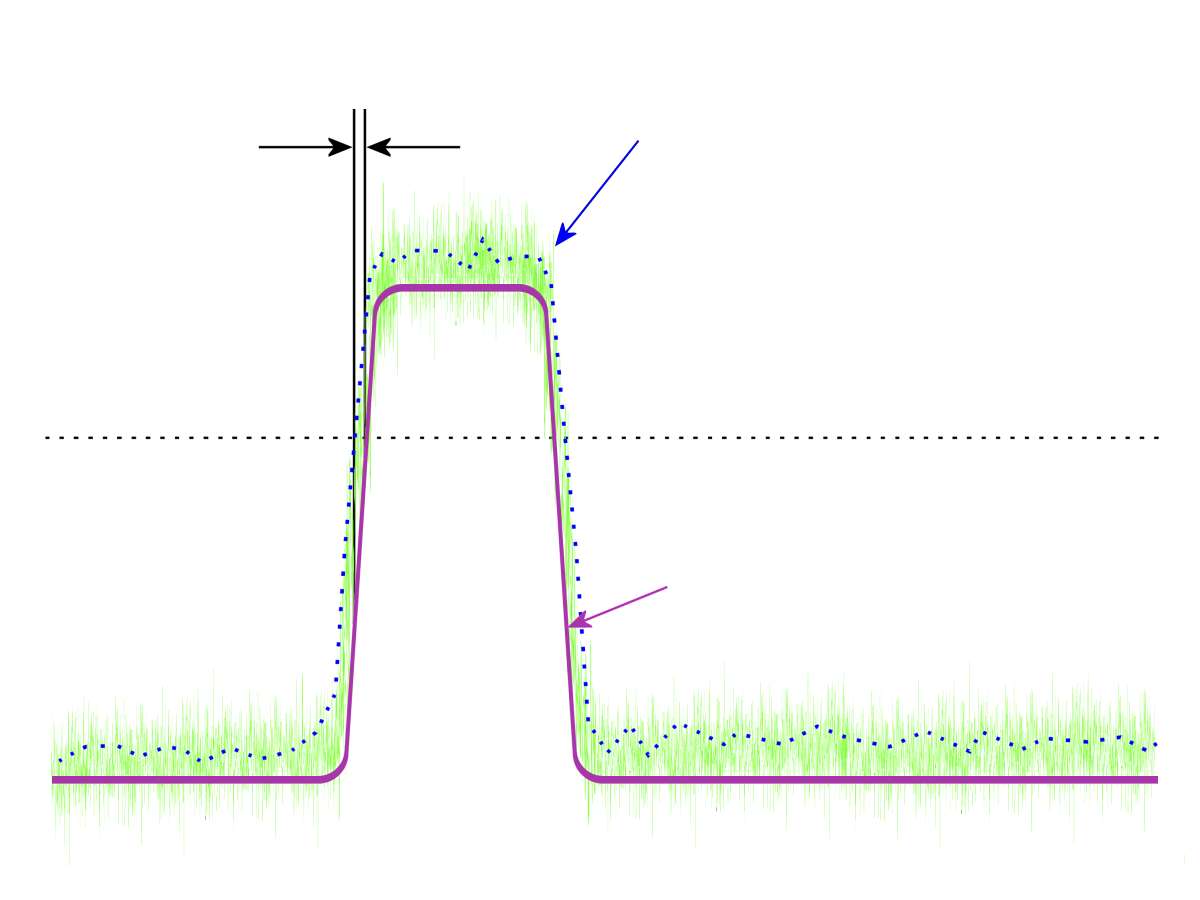
Figure 1: Falsification of the pulse edge due to superimposition of noise
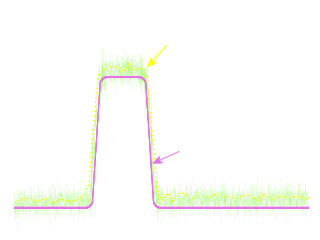
Figure 1: Falsification of the pulse edge due to superimposition of noise
Accuracy in range determination
The theoretical maximum accuracy with which a distance can be measured depends on the accuracy of the runtime measurement.
Random Measurement Error
A random error occurs with a pulse radar when the rising edge of the echo signal is distorted by noise. Since the pulse is always superimposed with noise during measurement and the pulse plus the noise is measured as amplitude, the pulse is also displayed larger than it is in reality. This shifts the pulse edge and causes a measurement error in the run-time measurement.
Figure 1 shows the influence of noise on the detectable edge of the echo pulse. The solid line (magenta) shows an almost ideal trapezoidal impulse with quite steep edges. This pulse cannot become quite rectangular because this would require an infinite bandwidth. The time is measured at a point determined by a threshold value, usually at 0.707 of the maximum voltage. However, this pulse is superimposed with the noise level (green). Only a voltage can be measured, which is formed by the sum of the instantaneous voltage of the pulse and the noise (dotted yellow line)(dotted blue line). This voltage exceeds the threshold value at an earlier time than the clean pulse. The difference is the random measurement error caused by the noise.[1]
If the duration of the pulse is known(which cannot be the case with primary radar but with secondary radar), then this random error can be reduced mathematically by simultaneous evaluation of the front and rear edges of the pulse.
Mathematical Context
As shown in Figure 1, the accuracy of the distance measurement depends essentially on the noise or better: the size of the noise in relation to the impulse. This quantity is described by the signal-to-noise ratio (SNR). The size of the noise itself also depends on the bandwidth. The slope of the pulse edge also depends on the bandwidth. For a signal-to-noise ratio of considerably higher than 1, the following relationship exists between these variables:[2]
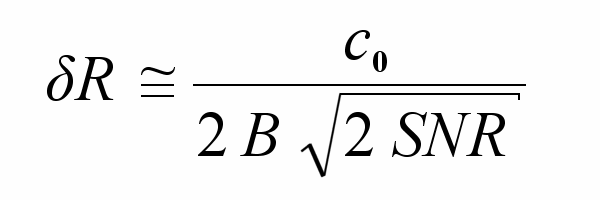
 δR = measuring error
δR = measuring errorc0 = speed of light
B = bandwidth
SNR = signal-to-noise ratio (1)
However, the bandwidth is also significant for the radars range resolution Sr = c0 / 2B. Thus, the maximum achievable accuracy can also be represented as a function of the radars range resolution:
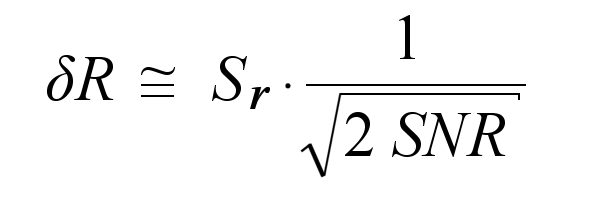
 (2)
(2)
From this, it can be seen that the maximum achievable accuracy in range determination must be considerably better than the range resolution.
Systematic Measurement Errors
With a pulse radar, the run time is generally measured from the rising edge of the transmit pulse to the rising edge of the echo signal. The accuracy of this measurement depends on the magnitude of the clock frequency for this time measurement. Measurement results between the cycles are not possible and generate a systematic measurement error. Practically, the accuracy depends on the size of the individual range-cells in signal processing. ICAO recommends[3] a range cell size of 1/128 NM, i.e. about 14.5 m, for air traffic control air surveillance radar, which corresponds to a time interval of almost 10 nanoseconds.
With a CW radar, the measurement of the phase shift of the received signal relative to the current phase of the transmitter may contain (albeit ambiguous) distance information.
The accuracy of an FMCW radar also depends on the transmitter, especially on the slope and linearity of the frequency drift.
Accuracy in Angle Measurement
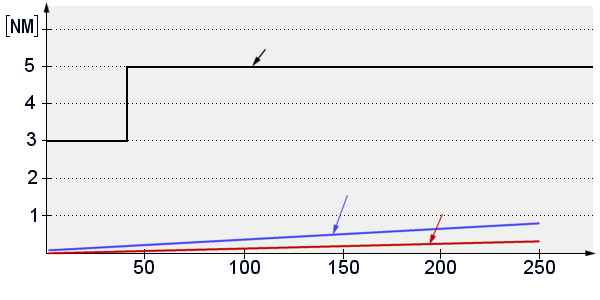
Figure 2: Dependence of the accuracy of the range
(Source: MIT Lincoln Laboratory)

Figure 2: Dependence of the angular accuracy of the range
(Source: MIT Lincoln Laboratory)
The accuracy of the angle measurement depends both on internal signal processing methods and on external conditions. Anomalous propagation conditions, which frequently occur due to changes in air pressure in height angle measurements, can in principle also occur in side angles and form a random error. However, more frequent systematic error sources occur internally.
For example, the angle determination by the sliding window is a rather inaccurate procedure. In practice, the half-width of the antenna is only divided by the number of quantizations of the method (e.g. 8 or 16 pulse periods) and thus results in a systematic error of the order of magnitude of up to one degree. Other correlation methods can also interpolate intermediate values and are therefore much more accurate. The best accuracy is currently achieved with the conical scan and the monopulse method.
How is a measurement performed?
The measurement is performed exactly as the measurement result is defined: the position measured by the radar is compared with the actual position of the target. In the case of an air surveillance radar, a test flight is carried out for this purpose, for example by FCS Flight Calibration Services GmbH. On board the Learjet 35 aircraft there is a recorder which records the current position of the aircraft using differential GPS with an accuracy of less than one meter. At the same time, the flight path is also recorded in the radar unit. Since both recorders are synchronized via the time base also provided by the GPS system, the positions can then be exactly compared with each other.
Statistical methods are also used for the calculation. Obvious erroneous measurements are excluded from the calculation because the systematic error of the radar unit is to be calculated. This does not mean, however, that many hits are necessary (perhaps to achieve a good value). If the radar uses monopulse technology, then a value is also determined for each pulse. If the radar determines the position using the Sliding Window method, then the respective value is determined according to the concrete number of hits required.
For good accuracy in distance determination, a stable and steep edge of the radar pulse is required. This steep pulse edge is often not visible when intrapulse modulation is used. But here it has to be said that the distance can only be measured after the pulse compression. At this point, the (now compressed) impulse is present again with a very good edge steepness.
The only condition for the measurement is that the radar operates in an interference-free environment. Interference-free means: the received echo signal is not superimposed by external interference signals. This also includes the noise level. A useful measurement is therefore only possible if the signal strength of the measured echo signal of the aircraft is much higher than the noise level. Finally, a flight calibration should detect possible additional systematic errors and not random errors.
Examples Given
Some results of radar units are indicated in the following table as example:
| radar unit | accuracy in bearing | accuracy in range | accuracy in height |
|---|---|---|---|
| BOR–A 550 | < ±0.3° | < 20 m | |
| LANZA | < ±0.14° | < 50 m | 340 m ≈ 1150 feet (at 100 NM) |
| GM 400 | < ±0,3° | < 50 m | 600 m ≈ 2000 feet (at 100 NM) |
| RRP–117 | < ±0,18° | < 463 m | 1000 m ≈ 3000 feet (at 100 NM) |
| MSSR-2000 | < ±0.049° | < 44.4 m | |
| STAR-2000 | < ±0.16° | < 60 m | |
| Variant | < ±0.25° | < 25 m |
Table 1: Examples
Referenzen:
- Merrill I. Skolnik: ''Introduction to Radar Systems'' McGraw-Hill Europe, 2001, ISBN 007-118189-x , S. 317, Topic 6.3 Theoretical Accuracy of Radar Measurements
- G. Richard Curry: ''Radar System Performance Modeling'' 2005, ISBN 978-1-58053-816-9, S.168
- ICAO Annex 10 - Volume 4. Aeronautical Telecommunications - Surveillance and Collision Avoidance Systems, Topic 4.3.2.1.3 Range and Bearing Accuracy, (Bundesamt für Zivilluftfahrt, Schweiz)
