Continuous Wave Radar
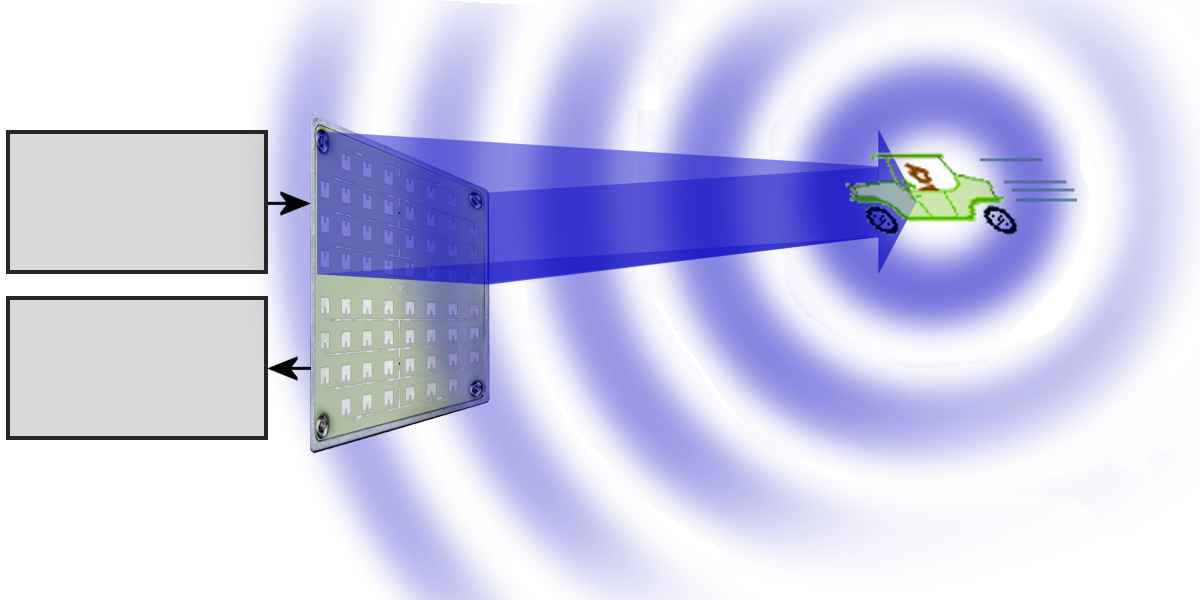
contains information about
the reflecting object
Figure 1: The continuous wave radar method often uses separate transmit and receive antennas. These are constructed on a double-sided printed circuit board.
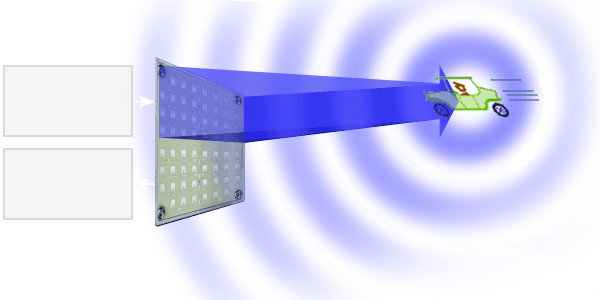
contains information about
the reflecting object
Figure 1: The continuous wave radar method often uses separate transmit and receive antennas. These are constructed on a double-sided printed circuit board.
Continuous Wave Radar
Continuous Wave Radar (CW radar) sets transmit a high-frequency signal continuously. The echo signal is received and processed permanently. One has to resolve two problems with this principle:
- prevent a direct connection of the transmitted energy into the receiver (feedback connection),
- assign the received echoes to a time system to be able to do run time measurements.
A direct connection of the transmitted energy into the receiver can be prevented by:
- the spatial separation of the transmitting antenna and the receiving antenna,
e.g. the aim is illuminated by a strong transmitter and the receiver is located in the
missile flying directly towards the aim;
- frequency dependent separation by the Doppler-frequency during the measurement of speeds.
A run time measurement isn't necessary for speed gauges, the actual range of the delinquent car doesn't have a consequence. If you need range information, then the time measurement can be realized by frequency modulation or phase keying of the transmitted power.
A CW-radar transmitting an unmodulated power can measure the speed only by using the Doppler- effect. It cannot measure a range and it cannot differentiate between two or more reflecting objects.
If an echo signal is received, this is initially only proof that there is an obstacle in the direction of propagation of the electromagnetic waves. The properties of the obstacle can be inferred from certain properties of the echo signal. For example, the strength of the echo signal depends on the size of the obstacle. Likewise, the strength of the echo signal is a sign of whether this obstacle is far away or near the radar. (Unfortunately, no measurement result is possible from this context, since the strength of the echo signal depends on too many factors.) A change in the frequency spectrum, on the other hand, is a safer feature for certain properties. Thus, harmonics of the transmission frequency can also occur during a reflection on certain materials. This is specifically exploited in a so-called “harmonic radar” in order to use these materials, which are incorporated into protective clothing, for example, to find people buried under the masses of snow in avalanche regions. However, the most commonly used changes in the spectrum are caused by the Doppler effect.
Doppler Radar
An unmodulated continuous wave radar emits a constant frequency with constant amplitude. The received echo signal either has exactly the same frequency, or the echo signal is shifted by the Doppler frequency (with a reflector moving at a radial velocity). CW radars that specialize in measuring this Doppler frequency are called Doppler radars.
A runtime measurement is not necessary at all with a Doppler radar for speed measurement, since no distance determination is carried out. If a runtime measurement is to be carried out, then a time reference of the received echo to the transmitted signal can be established by modulating the transmitted signal. This modulation, i.e. the actual time at which the transmitted signal changes in frequency or amplitude, can be registered in the receiver after the delay time and thus makes time measurement possible. Such modulation, however, results in other radar classes, which subsequently use completely different measurement principles (for example, frequency modulation: FMCW radar). Amplitude modulation at 100% modulation is also conceivable and would lead to a pulse radar. A radar that emits an unmodulated oscillation can only detect the speed of an object via the Doppler effect. It is not possible to determine distances or distinguish different targets in the same direction.
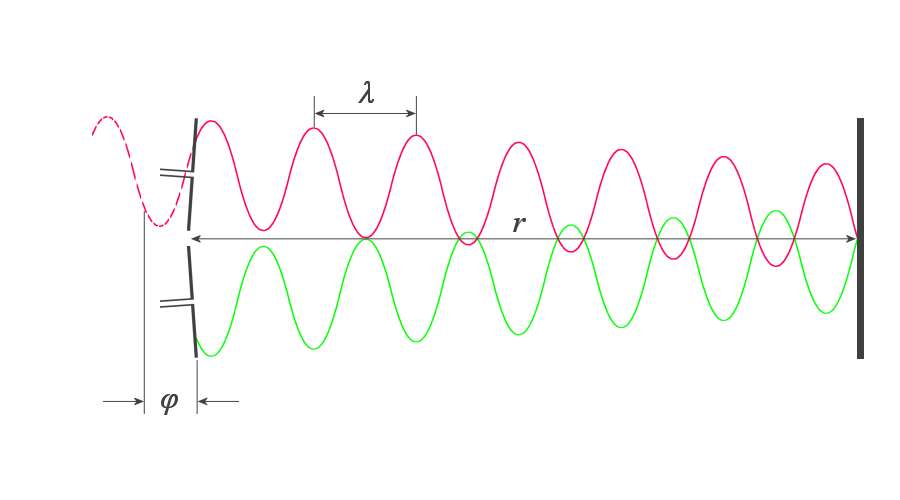
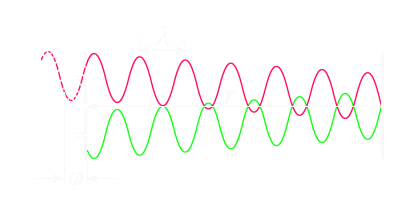
Figure 2: Phase difference
Function
The continuous wave radar evaluates the phase difference φ between the transmitted signal and the received signal. The magnitude of this phase difference is the ratio of the distance traveled by the electromagnetic wave to the wavelength of the transmitted signal, multiplied by the degree division of the full circle (2·π). The magnitude of this phase difference is the ratio of the wavelength of the transmitted signal to the distance traveled. If the distance to the reflector does not change, then it is constant and is calculated according to:
| φ = −2π | 2r | where | φ = Phase difference r = Distance of the reflector to the antenna λ = Wavelength of the transmitted signal |
(1) |
| λ |
The factor 2 to the distance r means that the signal has to pass through this distance twice (round trip). The minus sign is generated because a phase jump of 180° occurs during reflection. A direct calculation of a distance from this phase difference is not possible. For example, it would only be possible if the distance were between 0 and 2π (≙ φ<360°). From this distance, ambiguities arise due to the periodicity of the sine wave.
If the distance to the reflector is not constant but changes, for example, with a relatively constant speed to the transmitting antenna, the phase difference also changes as a function of time:
| φ(t) = −4π | r(t) | (2) |
| λ |
A time-dependent and constant change in the phase difference between two sinusoidal signals over the measurement period corresponds to a sinusoidal signal curve again. This can also be measured as a frequency: the Doppler frequency. In most cases, this is in the audio frequency range. At a constant transmission frequency, this Doppler frequency is proportional to the radial speed.

Amplifier
(Audio Processor)
Figure 3: Block diagram of a simple radar transceiver using direct downconversion
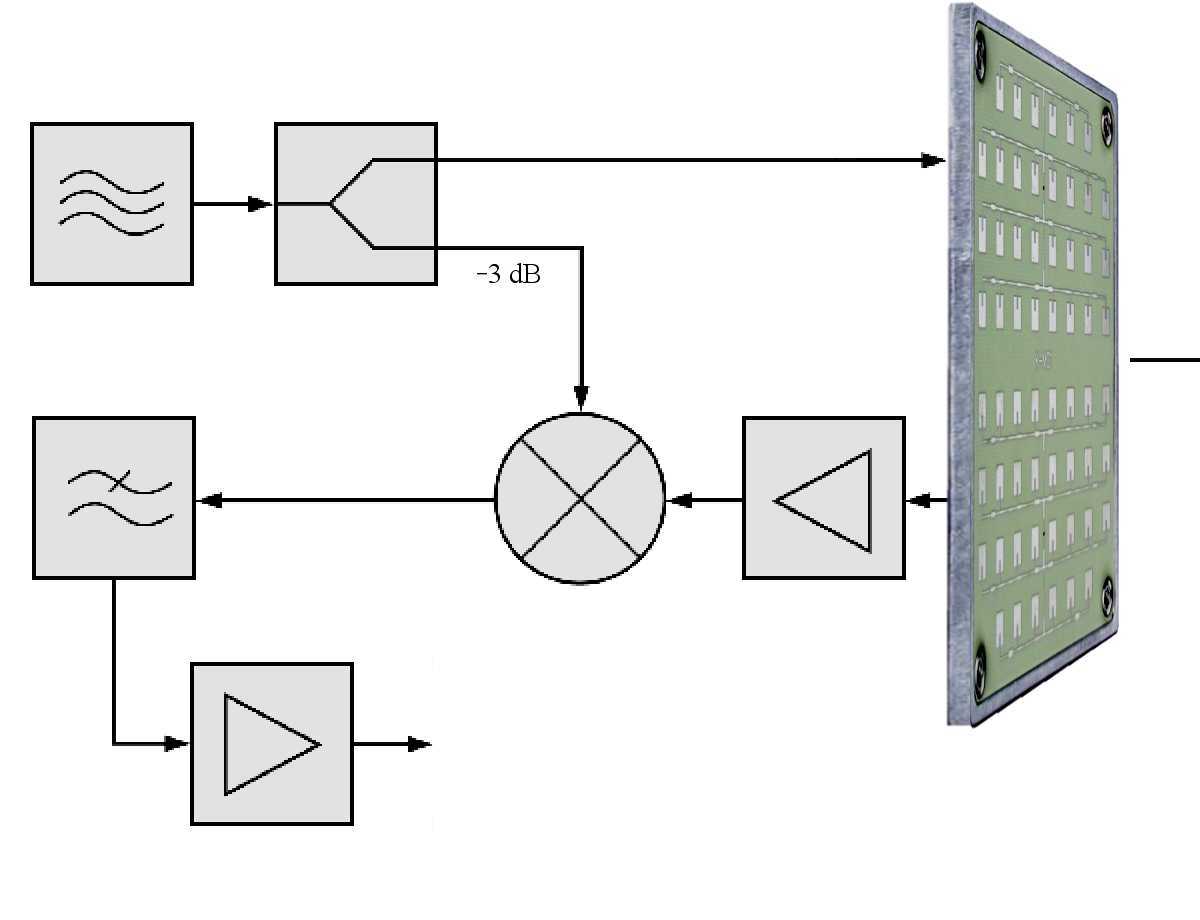
Amplifier
(Audio Processor)
Figure 3: Block diagram of a simple radar transceiver using direct downconversion

Amplifier
(Audio Processor)
Figure 3: Block diagram of a simple radar transceiver using direct downconversion (interactive picture)
Block diagram of CW radar
Direct conversion receiver
A Doppler radar for speed measurements is very simple.
The entire circuit of the transmitter and receiver can be manufactured with semiconductor components on a substrate as an integrated component.
This component is usually called a transceiver (a portmanteau of the words transmitter and receiver).
In many cases, this transceiver is already equipped with the required antennas.
Usually, these are
patch antennas
realized on a double-sided printed circuit board or (with larger bandwidths)
horn radiators.
With a receiver using direct conversion (or referred to a homodyne receiver), the echo signal is not converted into an intermediate frequency, but the high-frequency generated in the transmitter is also used directly for downconverting. The output signal of the mixing stage is then in the baseband, i.e. it's free of any carrier frequency. The mixers used usually require a local oscillator power of approximately 7 dBm in order to be able to downconvert the echo signal. Thus, the power of the HF generator is also set at 10 dBm. Since the power splitter has a minimum attenuation of -3 dB, the transmission power of at least 6 dBm is specified for the entire module. Although the output signal is now in the baseband, this output is still often referred to as “IF”, which suggests an intermediate frequency. However, the Doppler frequency is usually in the audible range. If this DC voltage is not blocked by coupling capacitors as high-pass filters then strong fixed target echoes occur at this output as DC voltage. Usually, such a circuit measure is also carried out in order to prevent signals that are generated by crosstalk from the transmitting antenna to the receiving antenna.
The maximum possible radial velocity v shall be calculated for a Doppler radar in K-Band, (λ≈ 12 mm) used as motion detector. How fast can a reflector be moved to process the echo signal with a stereo audio processor of a standard sound card? (fcut= 18 kHz = maximum fD)
In radar, the Doppler frequency is calculated according to:
| fD = | 2·v | fD = Doppler frequency [Hz] λ = the wavelength of the transmitted frequency [m] v = radial velocity [m/s] |
|
| λ |
This equation was converted according to v and the specified values were inserted:
| v = | λ · fD | = | 12 mm· 18 kHz | = 108 m/s ≈ 380 km/h |
| 2 | 2 |
With this configuration, a maximum of 380 km/h can be measured, which includes most cases of application for a simple motion detector.

Figure 4: Block diagram of a Doppler radar with heterodyne receiver
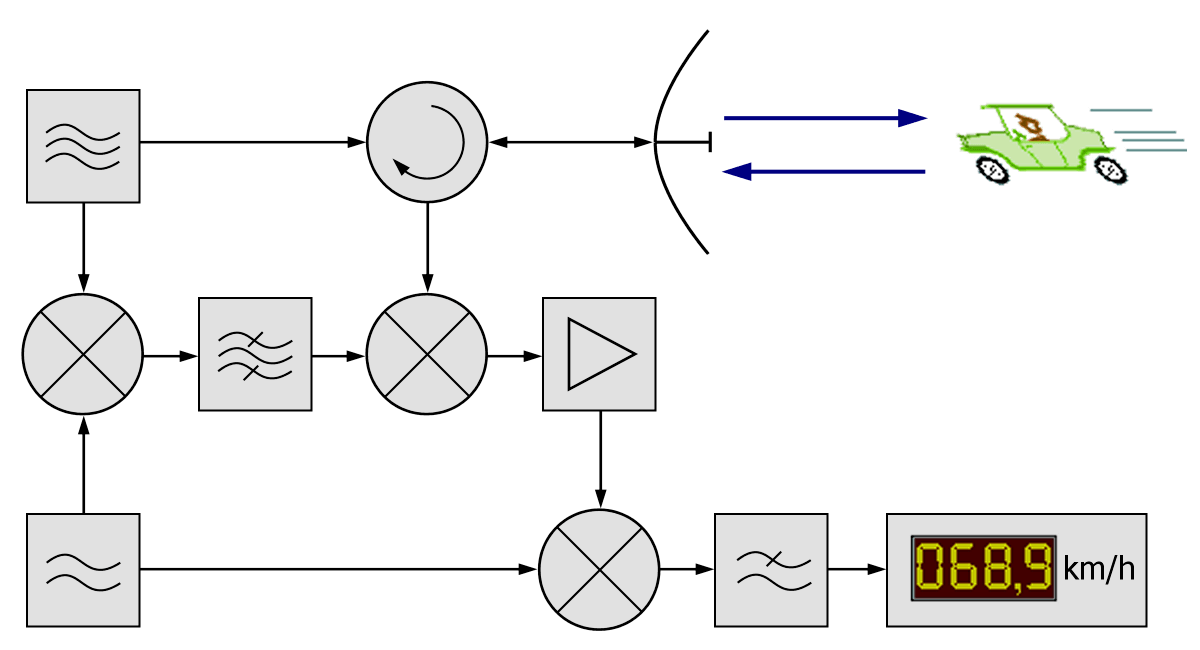
Figure 4: Block diagram of a Doppler radar with heterodyne receiver

Figure 4: Block diagram of a Doppler radar with heterodyne receiver (interactive picture)
Superheterodyne
By direct mixing, the sensitivity is limited.
Thus, the flicker noise of the mixer is given along with the output signal,
ie, the Doppler frequency is superimposed with a random distribution of low-frequency noise.
Very weak signals and low Doppler frequencies cannot be evaluated so often.
A significant improvement in sensitivity may provide at this point a superheterodyne receiver. The echo signals are converted first in a region which is well above the flicker noise. This flicker noise of the first mixer stage cannot pass through the bandpass filter of the intermediate frequency amplifier. Simultaneously, the echo signal is amplified by about 30…40 dB. Only in the second mixer, the echo signal is converted into the baseband. Since the amplified echo signal is now much larger than the flicker noise of the second mixing stage, this noise of the second mixer can be ignored.
In this example, only a single antenna for transmission and reception is used. The separation of the transmission energy of the received energy is performed with a circulator. The local oscillator frequency for the superheterodyne receiver is generated here by an upward mixing followed by a narrow-band filter. As an evaluation of the velocity, a counter is used herein. So that only a single reflective object may be displayed. (Which is usually the one with the highest amplitude.) If the radar observes a plurality of moving reflectors, then the overlapping Doppler frequencies need to be selected by a bank of filters, or a tunable filter. Nevertheless, several Doppler frequencies are possible to be measured, there is no way to attribute the simultaneously measured values to a particular object without any doubt.
Description of the modules in the block diagram
Calculation of radar range
In general, basic radar equation can also be used for continuous wave radar, since it is independent of the modulation type.
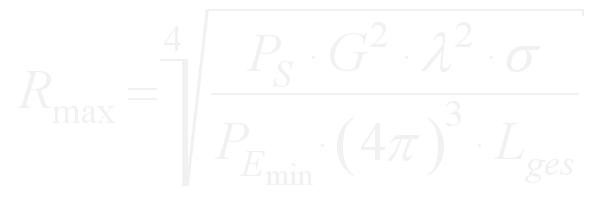
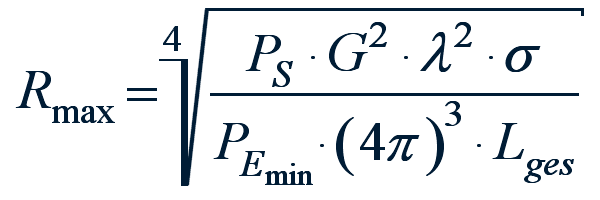
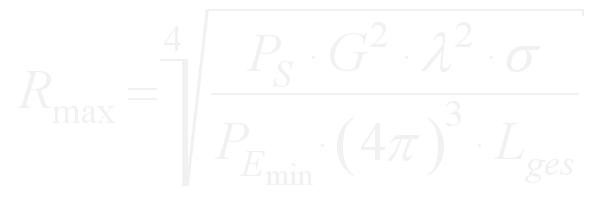
However, it must be borne in mind that the losses contained in the term Lges can also contain gains, for example through coherent integration.
Physicists would now point out that the decisive factor for the range of radar is not the transmitting power stated in the formula but the transmitted energy. This could previously be neglected in the derivation of the equation because the duration of the transmission pulse was assumed to be equal to the duration of the echo signal.
If the time duration of the demodulated echo signal differs from the time duration of the transmitted signal, these times must be put into relation and are multiplied as gain with the remaining values of the expression under the fourth root.
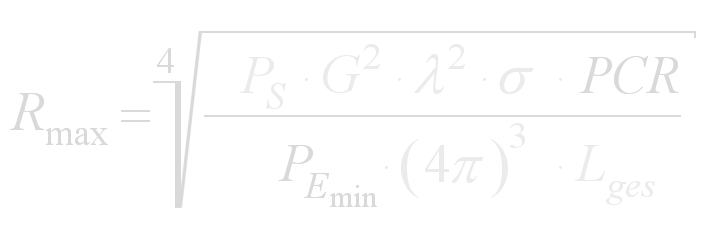
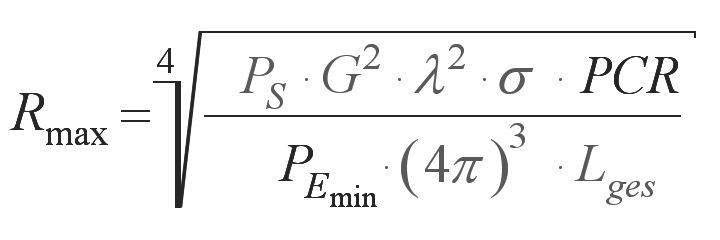
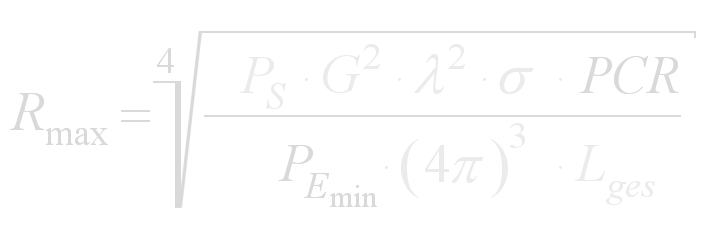
In radar, with intrapulse modulation, this gain is called pulse compression ratio (PCR) and depends on the transmitted bandwidth. This is illustrated by the fact that this transmitted modulation pattern can hardly be reproduced by random noise pulses so that the pulse compression filter can also detect targets far below the noise level.
A similar calculation can also be made for a continuous wave radar. Here, the integration gain can be calculated either with the dwell time in relation to the filter reaction time (CW-radar) or with the bandwidth (FMCW-radar).
Applications of unmodulated continuous wave radar

Figure 5: TRAFFIPAX SpeedoPhot, a German speed gauge (© 2000 ROBOT Visual Systems GmbH)
- Traffic control radar (Speed gauges)
- Speed gauges are very specialized CW-radars. A speed gauge uses the Doppler- frequency for measurement of the speed. Since the value of the Doppler- frequency depends on the wavelength, these radar sets use a very high frequency band. The figure shows the speed gauge „Traffipax Speedophot” produced by ROBOT Visual Systems GmbH. This radar uses a frequency of 24.125 gigahertz.
- It can measure the speed of the incoming and the outgoing traffic, from the right or left border of the street. The radar can be mounted in a car or on a tripod. The traffic offense can be substantiated by a high-resolution photo camera.
- Doppler radar motion sensor
Simple and inexpensive Doppler radar sensors with circuitry as shown in Figure 2 can trigger switching functions such as alarms or simply be used as a door opener or switch for lighting. - Motion monitoring
If the output of the mixer stage is DC coupled in Figure 2 (ie: in the mixing stage no inductive transformer or coupling capacitors are used) and the subsequent amplifiers are also all DC coupled, then this non-modulated continuous wave radar can monitors also distances to fixed targets with an accuracy in the order of about λ/16. Here no Doppler frequency is measured, it is compared the phase angle between the transmitted signal and the received signal. The way from the radar to the reflector and the way back is a multiple of the used wavelength. If this distance changes only by fractions of a millimeter, then the phase angle between the two signals also changes.
- The measuring range is ambiguous: how many full wavelengths must be additionally added to the measured fraction, this cannot be determined. The radar can monitor only changing to a previous value.
- By this measurement method, for example, non-contact monitoring of heart rate and respiratory activity of intensive care patients can be performed. The radar is aligned on the chest of the patient and monitors the distance to an accuracy of fractions of a millimeter. The changes of the phase angle between the transmitted signal and the received signal are displayed on an oscilloscope as a function of time. A connected to the radar computer counts the periodic changes and outputs the heart rate of the patient numerically. If no more changes are registered, an alarm is triggered.
- The absence of the minimum measuring range typical for pulse radar makes it possible to use this radar system design as a radio proximity fuse for missiles and artillery projectiles. The amplitude of the audible signal increases with the approach to the target, while the Doppler frequency decreases shortly before passing. Once the passband or low-pass of a filter has been reached, the radar proximity fuse triggers the warhead.