Calculation of Height
Figure 1: simple triangular relation between elevation and height
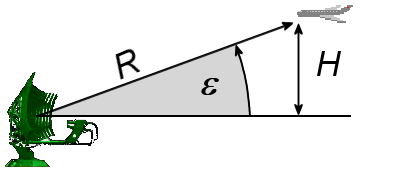
Figure 1: simple triangular relation between elevation and height

Figure 1: simple triangular relation between elevation and height
Calculation of Height
Target height (or altitude) is the distance of a target above the Earth's surface (height above ground). In equations, it is designated by the letter H. The height can be calculated from the target distance R and the elevation angle ε.
.en.print.png)
.en.png)
(1)
The values of distance R, the targets altitude H, and elevation angle ε we insert in the equation:
(2)
However, in relation to a real target, the target height cannot be calculated so easily because the electromagnetic waves in the atmosphere are refracted at the air layer boundaries (different densities) and the Earth's surface is curved. Because the calculation of the target height is not only a trigonometric calculation but also the Earth's curvature must be considered, which is valid for the location. Both factors are compensated by complex equations in radar systems with integrated height calculation. A frequently used approximation is:
.print.png)
.png)
(3)
- R = targets slant range
- ε = measured elevation angle
- re = Earth's radius (about 6370 km)
- (This equation is an approximation only!)

Figure 2: relation between elevation and height under consideration of the Earth bend
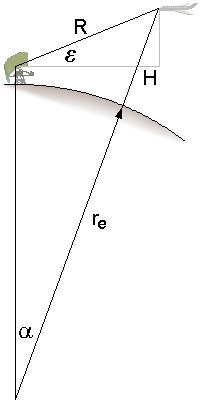
Figure 2: relation between elevation and height under consideration of the Earth bend

Figure 2: relation between elevation and height under consideration of the Earth bend
The solution can be seen in Figure 2. A triangle between the points: Center of the Earth, the radar site and the location of the target, whose sides are represented by the cosine theorem and thus by the equation:
(4)
Calculation of the Down Range
Under the assumption that the Earth is a sphere, the section of the circumference of the Earth can be calculated with help of a simple ratio from the complete circumference of the Earth from the angle α:
(5)
This segment of the circumference of the Earth can be considered an approximation of the actual topographical range (here though still without considering refraction).
Height Calculation with the Influence of Refraction
In practice, however, the propagation of electromagnetic waves is also subject to a refraction, this means, the transmitted beam of the radar unit isn't a straight side of this triangle but this side is also bent and it depends on:
- the transmitted wavelength,
- the barometric pressure,
- the air temperature and
- the atmospheric humidity.
The Earth radius can be multiplied with a factor for an approximation of the influence of the refraction. An equivalent Earth radius of 4/3 ·re ≈ 8500 km is often used and can additionally modified by a manual input of a correction factor to consider temporarily changed weather conditions or changed barometric pressure.
E.G. the following equation is used to calculate the height into the height-finder PRV–16
06.png) (6)
(6)
meaning at this:
- height without including the Earth's radius
- term including the Earth's equivalent radius (about 8500 km)
- term including the refraction into the atmosphere
- term including the refraction’s dependence on temperature.
